Irrational Numbers

Irrational numbers are real numbers that cannot be represented as simple fractions. An irrational number cannot be represented as a ratio, such as p / q, where p and q are integers, and q is not equal to zero. It is a contradiction of rational numbers. Irrational numbers are usually expressed as R/Q, where 'set minus' is denoted by a backward slash. It can also be expressed as R - Q, which states the difference between a set of real numbers and a set of rational numbers.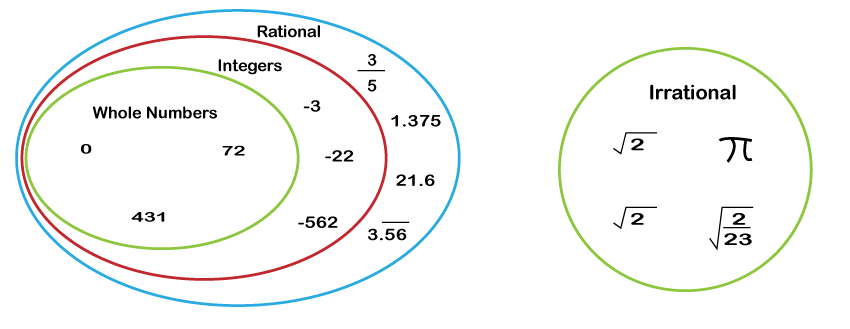

$Irrational Numbers$

The calculations that are based on these numbers are a bit complicated. For example √5, √11, √21, etc., are irrational. If such numbers are used in arithmetic operations, then first, we need to evaluate the values under the root. Now let us discuss its definition, lists of irrational numbers, how to find them, etc., in this article.

What are Irrational Numbers?

An irrational is defined as a real number that cannot be expressed as a ratio of integers, for example, root 2 is an irrational number. No irrational number could be expressed in the form of a ratio, such as p/q, where p and q are integers, and q is not equal to zero. Again, Irrational number has neither terminating recurring numbers.

Meaning of irrational: The meaning of irrational is not having a ratio or no ratio can be written for that number. In other words, we can say that irrational numbers cannot be represented as the ratio of two integers.

What are examples of Irrational Numbers?

The common examples of irrational numbers are pi ( pi(π=3⋅14159265…), √2, √3, √5, Euler's number (e = 2⋅718281…..), 2.010010001…., etc.

How do we know a number is irrational?

The real numbers which cannot be expressed in the form of p / q, where p and q are integers and q are not equal to zero are known as irrational numbers. For example, root 6 and root 7 are irrational numbers. Whereas if a number can be represented in the form of p / q, such that, p and q are integers and q is not equal to zero is known as a rational number.

Is pi an Irrational Number?

Yes, Pi (π) is an irrational number because it is neither terminating nor repeating decimals. Also, Pi is not equal to 22 / 7 as 22 / 7 is a rational number while we know pi is an irrational number. The value of π is 3.141592653589………..

Note- Rational numbers (Q) and Irrational numbers (P or Q' ) are always alternate with each other.

Therefore, 22/7 ≠ π but they are alternate or next to each other.

Symbol of an Irrational Number

Generally, Symbol 'P' is used to represent the irrational number. Also, since irrational numbers are defined negatively, the set of real numbers ( R ) that are not the rational number ( Q ) is called an irrational number. The symbol P is often used because of its association with real and rational. Because of the alphabetic sequence P, Q, R. But mostly, it is represented using the set difference of the real minus rationals, in a way R-Q or R/Q.

Properties of Irrational Numbers

As we know irrational numbers are the subsets of real numbers, irrational numbers will obey all the properties of the real number system. The following are the properties of irrational numbers:

If we add a rational number and an irrational number, we will get an irrational number. For example, let us assume that x is an irrational number and y is a rational number, and the addition of both the numbers x + y gives an irrational number w.
If we multiply an irrational number with any nonzero rational number results in an irrational number. Let us assume that if my = z is irrational, then x = z/y is rational, contradicting the assumption that x is irrational. Thus, the product XY must be irrational.
The least common multiple ( LCM ) of any two irrational numbers may or may not exist.
The addition or the multiplication of twoo irrational numbers may be rational; for example root 2* root 2 = 2. Here, root 2 is an irrational number. If it is multiplied twice, then the final product obtained is a rational number. i.e., 2.
The set of Irrational numbers is not closed under the multiplication process, unlike the set of rational numbers.

List of Irrational Numbers

The famous irrational numbers consist of Pi, Euler's number, and the Golden ratio. Also, many square root numbers and cube root numbers are also irrational, but not all of them. For example root 5 is an irrational number, but root 4 is a rational number. Because 4 is a perfect square, such as 4 = 2 x 2 and √4 = 2, which is a rational number. It should be noted that there are infinite irrational numbers between any two real numbers. For example, say 3 and 4, there are infinitely many irrational numbers between 3 and 4. Now, let us look at famous irrational numbers' values.

Note - √prime number always gives an irrational number.

Pi, π	3.14159265358979…
Euler's Number, e	2.71828182845904…
Golden ratio, φ	1.61803398874989….

Are Irrational Numbers real numbers?

In mathematics, all irrational numbers are considered real numbers, which should not be rational numbers. It means irrational numbers cannot be expressed as the ratio of two numbers. For example, the square roots that are not perfect will always result in an irrational number.

Let's discuss the sum and product of two Irrational Numbers

Product of two Irrational numbers:

Statement: The product of two irrational numbers can be anything either rational or irrational.

For example, root 3 is an irrational number but when its multiplied with root 3 again, the product that we get i.e., 3 is a rational number.

(i.e.,) √3 x √3 = 3

We know that a pi is also an irrational number, but if pi is multiplied by pi, the result is pi square, which is also an irrational number.

(i.e..) π x π = π²

It should be noted that multiplying the two irrational numbers, may result in an irrational number or a rational number.

Sum of two Irrational Numbers:

Statement: The sum of two irrational numbers may be rational or irrational.

Like the product of two irrational numbers, the sum of two irrational numbers will also result in a rational or irrational number.

Let's discuss with an example, if we add two irrational numbers, say 3√2+ 4√3, a sum is an irrational number.

But, let us consider another example, (3+4√2) + (-4√2 ), the sum is 3, which is a rational number.

So, we should be very careful while adding and multiplying two irrational numbers, because it might result in an irrational number or a rational number.

Definition and proof of Irrational Number theorem

Theorem: Given p is a prime number and a² is divisible by p,(where a is any positive integer), then it can be concluded that p also divides a.

Proof: Using the Fundamental Theorem of Arithmetic, the positive integer can be expressed in the form of the product of its primes as:

a = p₁ × p₂× p_3………..× p_{n …..(1)}

Where, p_1, p_2,p₃,_……,p_n represent all the prime factors of a.

Squaring both the sides of equation (1),

a² = ( p₁ × p₂× p_3………..× p_{n) (} p₁ × p₂ × p₃……….. × p_n)

⇒a² = (p₁)² × (p₂)²× (^p3)^2………..× (p_n)²

According to the Fundamental Theorem of Arithmetic, the prime factorization of a natural number is unique, except for the order of its factors.

The only prime factors of a² are p₁, p_2, p_{3………..,} p_n. If p is a prime number and a factor of a², then p is one of p₁, p_{2 ,} p_{3………..,} p_n. So, p will also be a factor of a.

Hence, if a² is divisible by p, then p also divides a.

Now, using this theorem, we can prove that √ 2 is irrational.

How to Find an Irrational Number?

Let us find the irrational numbers between 2 and 3.

We know the square root of 4 is 2; √4 =2

and the square root of 9 is 3; √9 = 3

Therefore, the number of irrational numbers between 2 and 3 are √5, √6, √7, and √8, as these are not perfect squares and cannot be simplified further. Similarly, you can also find irrational numbers, between any other two perfect square numbers.

Another Case:

Let us assume a case of √2. Now, how can we find if √2 is an irrational number?

Suppose √2 is a rational number. Then, by the definition of rational numbers, it can be written that,

√ 2 =p/q …….(1)

Where p and q are co-prime integers and q ≠ 0 (Co-prime numbers are those numbers whose common factor is 1).

Squaring both sides of equation (1), we have

2 = p²/q²

⇒ p² = 2 q ² ………. (2)

From the theorem stated above, if 2 is a prime factor of p², then 2 is also a prime factor of p.

So, p= 2 × c, where c is an integer.

Substituting this value of p in equation (3), we have

(2c)² = 2 q ²

⇒ q² = 2c ²

From this, we can understand that 2 is the prime factor of q square also. Again from the theorem, it can be said that 2 is also a prime factor of q.

According to the assumption that we made initially, p and q are co-primes but the result obtained above contradicts this assumption as p and q have 2 as a common prime factor other than 1. This contradiction arose due to the incorrect assumption that root 2 is rational.

By this we can prove that root 2 is irrational.

Similarly, we can justify the statement discussed in the beginning that if p is a prime number. Then root p is an irrational number. Similarly, it can be proved that for any prime number p, root p is irrational.